LeetCode-in-All

141. Linked List Cycle

Easy

Given head, the head of a linked list, determine if the linked list has a cycle in it.

There is a cycle in a linked list if there is some node in the list that can be reached again by continuously following the next pointer. Internally, pos is used to denote the index of the node that tail’s next pointer is connected to. Note that pos is not passed as a parameter.

Return true if there is a cycle in the linked list. Otherwise, return false.

Example 1:

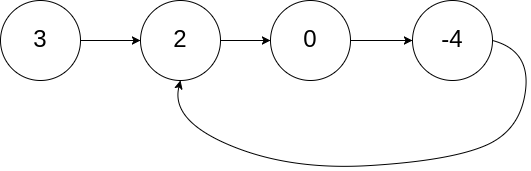
Input: head = [3,2,0,-4], pos = 1

Output: true

Explanation: There is a cycle in the linked list, where the tail connects to the 1st node (0-indexed).

Example 2:

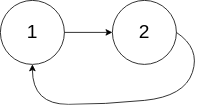
Input: head = [1,2], pos = 0

Output: true

Explanation: There is a cycle in the linked list, where the tail connects to the 0th node.

Example 3:

Input: head = [1], pos = -1

Output: false

Explanation: There is no cycle in the linked list.

Constraints:

• The number of the nodes in the list is in the range [0, 104].
• -105 <= Node.val <= 105
• pos is -1 or a valid index in the linked-list.

Follow up: Can you solve it using O(1) (i.e. constant) memory?

To solve the “Linked List Cycle” problem, we can use the Floyd’s Tortoise and Hare algorithm. This algorithm uses two pointers moving at different speeds to detect if a cycle exists in the linked list. Here’s a step-by-step guide and the Swift implementation of the solution.

Steps to Solve the Problem

1. Initialization:
   • Define two pointers, slow and fast, both starting at the head of the linked list.
2. Traversal and Cycle Detection:
   • Move the slow pointer one step at a time.
   • Move the fast pointer two steps at a time.
   • If there is a cycle, the fast pointer will eventually meet the slow pointer.
   • If the fast pointer reaches the end of the list (i.e., fast or fast.next becomes nil), there is no cycle in the linked list.
3. Return Result:
   • If the fast pointer meets the slow pointer, return true indicating a cycle.
   • If the fast pointer reaches the end, return false.

Swift Implementation

Here’s the implementation of the Solution class using the Floyd’s Tortoise and Hare algorithm:

// Definition for singly-linked list.
class ListNode {
    var val: Int
    var next: ListNode?
    init(_ val: Int) {
        self.val = val
        self.next = nil
    }
}

class Solution {
    func hasCycle(_ head: ListNode?) -> Bool {
        // Initialize slow and fast pointers
        var slow = head
        var fast = head
        
        // Traverse the linked list
        while fast != nil && fast?.next != nil {
            slow = slow?.next         // Move slow pointer one step
            fast = fast?.next?.next   // Move fast pointer two steps
            
            // Check if slow and fast pointers meet
            if slow === fast {
                return true
            }
        }
        
        // If we reach here, there is no cycle
        return false
    }
}

Explanation of the Swift Code

1. Initialization:
   • The ListNode class defines the structure of a node in the linked list.
   • The Solution class contains the hasCycle method which checks for a cycle.
2. Traversal and Cycle Detection:
   • The while loop continues as long as fast and fast?.next are not nil.
   • The slow pointer is moved one step (slow = slow?.next).
   • The fast pointer is moved two steps (fast = fast?.next?.next).
3. Cycle Detection:
   • If the slow pointer and fast pointer meet (if slow === fast), it indicates there is a cycle, and the method returns true.
   • If the loop exits because fast or fast?.next is nil, it means there is no cycle, and the method returns false.

This approach ensures that we use constant space (O(1)) while efficiently detecting the presence of a cycle in the linked list.

This site is open source. Improve this page.